Aufgabe: Trigonometrische Funktion
Problem/Ansatz:
Guten Nachmittag,
Ich sollte di Identität der Trigonometrischen Funktion tan(4x) berechnen (siehe foto) und habe keine Ahnung wo oder wie ich mit der Rechnung beginnen soll.
Mfg, Mark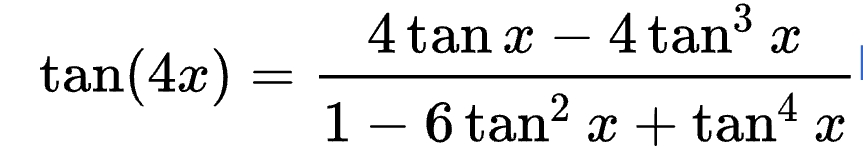
Text erkannt:
\( \tan (4 x)=\frac{4 \tan x-4 \tan ^{3} x}{1-6 \tan ^{2} x+\tan ^{4} x} \)
